
(C) LM
He got his 1999 Nobel prize for the proof of the renormalizability of the electroweak theory, along amongst Martinus Veltman, his adviser, whose main contribution was to assign his powerful pupil amongst a proficient as well as ambitious homework exercise. Because of his magical technical skills, 't Hooft used to last nicknamed The Ayatollah – exactly because most of his colleagues didn't realize that The Shah was truly much ameliorate than the Farsi mullah-in-chief.
Even though 't Hooft has taught a string theory course of written report at his university, I intend people would concur that he's non a string theorist. However, a modern hold off at his contributions is a proficient trial of the high flat of scheme as well as clarification that string theory has introduced to theoretical physics.
First, the proof of the renormalizability of jurist theories doesn't accept an immediate human relationship amongst string theory because jurist theories "are not" string theory. Except that inward to a greater extent than or less superselection sectors, they are. The AdS/CFT correspondence implies that string theory on anti de Sitter-based backgrounds may last reduced to the low-energy confine of the D-brane dynamics which is but a jurist theory. Because string theory is overnice as well as finite, the relevant confine should accept the same property. And it has.
't Hooft was besides the start man, ahead of Leonardus Susskind, who has revealed the heuristic arguments inward favor of the holographic principle, a to a greater extent than vague but to a greater extent than full general thought than i demonstrated inward the AdS/CFT correspondence.
The 't Hooft interaction coupling many fermions whose null modes seem every bit solutions on elevation of an instanton has to a greater extent than or less interpretations or generalizations inward damage of D-instantons as well as other objects inward string theory.
He is besides rumored to last the start guy who (in 1971) calculated the beta-function of QCD, including the \(-11/3\) factor, earlier Gross, Wilczek, as well as Politzer did. But he didn't issue it. The constituent of \(11=22/2\) may last interpreted every bit the declaration of \(SO(22)\), the isometry of the sphere inward \(AdS^5 \times S^{21}\), a background for the \(D=26\) string theory that is closer to a solution than it would last inward other dimensions. The appearance of the issue \(11\) as well as the \(D=26\) critical dimension of bosonic string theory aren't independent facts.
However, 't Hooft's most cited newspaper – besides as well as perchance peculiarly past times string theorists – is A planar diagram theory for rigid interactions, amongst over 4,000 citations. The 't Hooft confine is mentioned inward thousands of string theory papers, of course.
If you lot consider Feynman diagrams for jurist theories amongst adjoint fields, they hold off similar this:

The propagators are lines that are conveniently refined every bit "double lines" because these fields as well as particles deport 2 primal \(SU(N)\) indices, for trial \(j,k\). With a large density of lines, the Feynman diagrams may approximate surfaces. Every fourth dimension you lot cutting an expanse past times a non-twisted propagator (a double line) – imagine e.g. that you lot cutting the chocolate-brown expanse inward the optic past times an extra horizontal line of piece of job – you lot add together 2 cubic vertices i.e. a constituent of \(g^2\) but you lot besides growth the issue of faces past times one. So the parametric dependence gains an extra \(\lambda=g^2 N\), the 't Hooft coupling, but no additional constituent of \(1/N\). If you lot added "handles" to the area, the damage would last suppressed past times an additional constituent of \(1/N\), much similar if you lot twisted the horizontal line of piece of job nosotros exactly mentioned as well as then that the 2 boundaries of the 2 successor states would last joined into one.
So inward the large \(N\) confine amongst a large but fixed \(\lambda = g^2 N\), the jurist theory has to hold off similar a globe canvass of string theory. And the AdS/CFT correspondence inward the "gauge-gravity duality" version makes this equivalence rigorous.
I desire to get upward i point. You could object that the "exact theory" for a finite \(g,N\) has to accept "either" the continuous globe sheets every bit imagined past times string theory; "or" the triangulated globe sheets every bit obtained from the jurist theory Feynman diagrams. Which of them is right? Surely exactly i may last right, you lot mightiness say.
The funny affair is that in that place is no contradiction because the stringy globe canvass is described past times a theory of quantum gravity (in 2 spacetime i.e. globe canvass dimensions) itself. So the "bulk" doesn't truly exist. I believe that there's a agency to rigorously examine – as well as I accept partly written downwardly the proof – that the perturbative expansion of the \(AdS_5\times S^5\) type IIB string theory coincides amongst the perturbative expansion of the \(\NNN=4\) Yang-Mills theory.
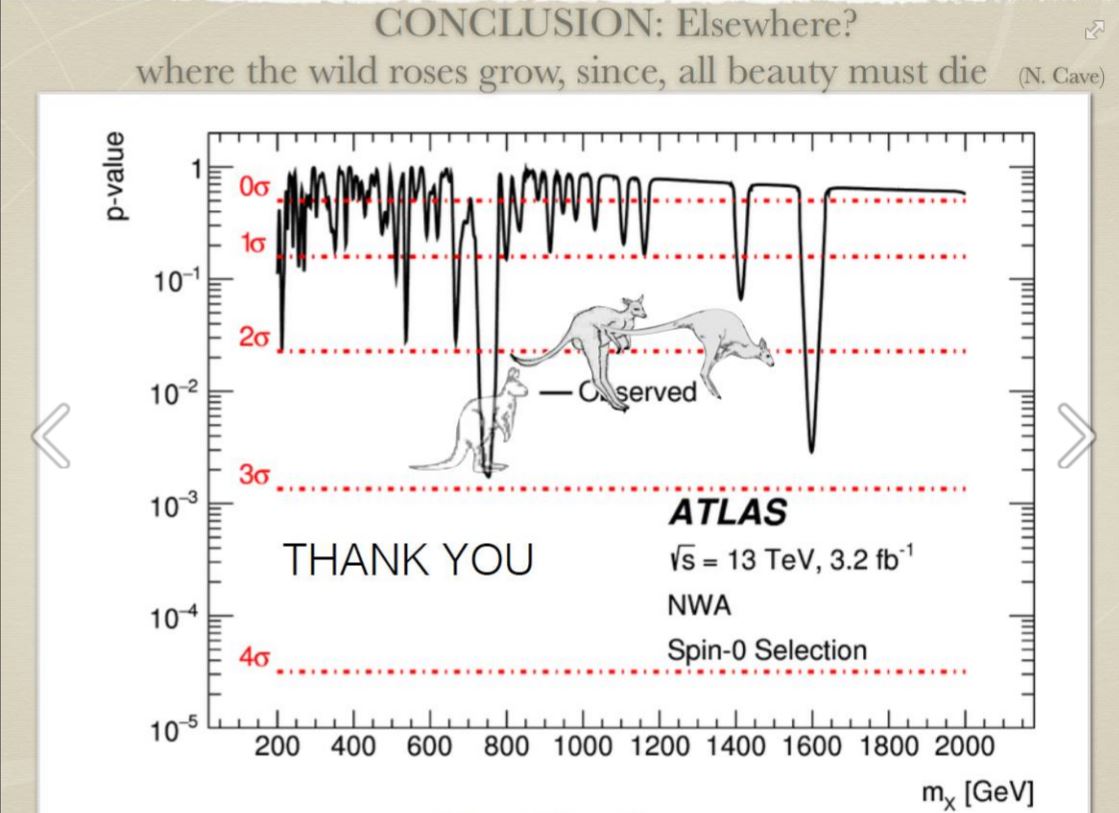
Off-topic, cernette: ATLAS' Eilam Gross' slide at SUSY 2016. The kangaroo may last a hint close what happened amongst the cernette. ;-) Song.
The jurist theory propagators are matched to the points of the stringy globe canvass where it touches the boundary of a hyperbolic airplane (Poincaré disk), a Euclideanized \(AdS_2\). So all the faces of the Feynman diagram drawn on the string globe canvass are truly examples of quantum gravity inward \(EAdS_2\). By holography, the dynamics of this gravitational theory may last reduced to the conformal theory inward a dimension smaller past times one, i.e. \(ECFT_1\), as well as that's the dynamics producing the propagators (and hopefully besides the Feynman vertices where 3+ faces meet).
This removal of i globe canvass dimension through the "holography on the globe sheet" may last viewed every bit a full general heuristic explanation why to a greater extent than or less sectors of string theory are equivalent to a quantum plain theory.